The Banach-Tarski Theorem

The Banach-Tarski paradox posits that one can take a solid sphere, split it into a finite number of disjoint subsets, and then reassemble those subsets into two identical copies of the original sphere. This notion challenges my intuition and seems to defy common sense, as it implies the ability to create something from nothing, contradicting the conservation of matter and energy.

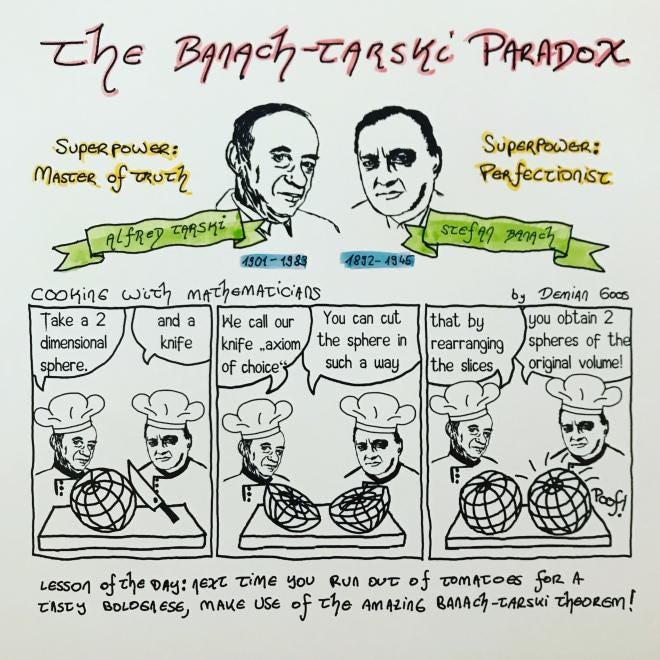
The paradox arises due to the non-measurable characteristics of certain sets, which means they lack a clearly defined measure like length, area, or volume. The involved sets possess an infinite “size” in a certain sense, allowing for unexpected manipulations like “stretching” or “duplicating.” However, the explanation remains somewhat elusive. Let’s explore further.

The foundation of the paradox begins with a solid sphere in three-dimensional space, which is a continuous and infinitely divisible object. Through mathematical principles from measure theory, such as Lebesgue measure, one can define the size of a subset of the sphere. This measure intuitively represents the “size” or “volume” of the set, although its precise definition is more abstract and technical.

The paradox emerges from the existence of “paradoxical” subsets of the sphere, which can be broken down into a finite number of pieces and reassembled into two spheres identical in size to the original. This notion seems implausible since the total quantity of matter and energy should remain unchanged, and cannot be created or destroyed through any physical means.

To grasp how this paradox can manifest, we must delve into the properties of these paradoxical sets. They exhibit several remarkable traits:

1. Non-measurable: These sets cannot be assigned a well-defined measure using conventional measure theory. They possess an infinite measure or “size,” leading to unexpected behaviors.
2. Non-constructive: They cannot be explicitly defined or constructed using standard mathematical operations. Instead, they rely on abstract concepts from set theory and topology, such as the axiom of choice.
3. Self-similar: These sets display a fractal-like structure, where smaller copies can be found at various scales. This characteristic enables the decomposition of the set into a finite number of pieces that can be rearranged to form two spheres.

Understanding the Banach-Tarski Paradox

The paradoxical sets used to demonstrate the Banach-Tarski paradox are derived through a group-theoretic method known as the Hahn-Banach extension theorem. This technique permits certain linear transformations to be extended to larger spaces, involving advanced concepts from functional analysis and measure theory.

The implications of the Banach-Tarski paradox are profound for the foundations of mathematics, including measure theory, group theory, and even quantum mechanics. It reveals that our intuitive understanding of objects in three-dimensional space can be misleading and counterintuitive. However, it's essential to note that these insights apply solely to idealized mathematical constructs and do not extend to real-world objects or physical processes. So, if you're considering creating something from nothing at home, consider this a gentle warning!